12 of 12, July 2017

This is the post in which I attend a maths teachers’ conference and demonstrate my incapacity to identify and stop at a count of 12. That’s irony for you.

Last week I finally got around to getting myself a “professional” Twitter account for my work identity, so that I can join the large number of maths education people blurting 140 character messages at the universe every so often. Today I thought I would combine tweeting things that I learned or found interesting at the conference with my 12 of 12 project, by picking “mathsy stuff” as the theme for this month’s 12 of 12, and tweeting the photos on Twitter as well as using them here. I got somewhat carried away however, and so there were 14 tweets/photos, and I’ve added one extra here to help explain one of them. To make matters worse for you, dear blog reader, I’m going to add more explanation here than I could manage on Twitter.

Overnight there had been discussion on Twitter about a problem that had been presented at the conference: take a square with a 12cm side (shown on the right in brown below), adjacent to a square of unspecified side length (in green), and consider the triangle shown in blue. What is its area? It turns out that, no matter the size of the green square, the triangle always has an area of 72. Last night, after solving the problem myself, I had made a Geogebra file of the problem that allows you to change the size of the green square, and see the triangle change shape but remain at a constant area. I was looking at it again this morning so that I could send a copy to people who had been discussing the problem.

For many of my tweets, I just posted the photo and posed a question. For the photo below, noticed as we walked to the conference venue, I asked (with no expectation that anyone would actually answer, an expectation I continue here!), what proportion the light coloured tile takes up in relation to the ochre brick.

As we arrived at the convention centre, I noticed this array of tactile safety dots on the steps to the conference convention centre. Arrays mean multiplicative thinking, a key area of fundamental maths. How many dots are shown? Can you estimate the size of each dot? Could you work out how many there are in the 10m strip that runs along the front of the centre?

Just before I went inside I took a photo of one of the many winter-denuded skeletal trees, making a natural fractal shape to enjoy. In a fitting connection, today’s keynote included the nice and more regular numerical fractal that arises in Pascal’s triangle if you colour it in according to whether the numbers are odd or even.

In a somewhat larger magnitude repeat of something I did for June’s 12 of 12 I took a photo of the main hall of the conference centre. I counted the number of seats (how?) and then, once everyone had turned up some time after the photo below was taken, I estimated that there were 264 people attending the keynote (how do you think I estimated?). I can now ask, how many ways can you arrange those 264 people on the 384 seats?

It would have taken five Twitter posts to give all 629 digits of the incomprehensibly gargantuan answer, but here they are:

3020404115417039853269377279002361651511851672054440088146527085604384245204285814139004128 6517820540828494897628003991370579249357239100547927916454805602272429772891593405093785441 8261647941653912698727420964016034449605436084101824432740115339520555291133664392033451257 3341871713243607194544423354253191919960278326915183881918709488591702868170426458846296294 0614269058722905203851628714756144702048888482056013009065847299888972814848005759408527315 6034503246463283097454032504514249322311203398682758664117248955722165681020871802390223341 47877537732100096000000000000000000000000000000000000000000000000000000000000000000

[I’d got 384 from counting that there were 16 rows of 24 chairs (in 3 sets of 8 columns), and I’d calculated 24×16  as (20+4)x(20-4) = 20^2 – 4^2 = 400-16 = 384. Just before the end of the lecture I took a few eye-balled samples of sets of 8 seats from around the room to ascertain that there were 5 to 6 people per 8 seats, so I took there to be 5.5/8 people per chair, which I turned into the equivalent-fraction 11/16. This made calculating 11/16 x 24 x 16 an easy calculation, and I used the quick way to multiply 11 by 24 to get 264.]

The keynote was a thoroughly enjoyable tour de force of number patterns in Pascal’s Triangle and even the humble multiplication tables. I think I’d seen how the Fibonacci numbers can be found inside Pascal’s Triangle but I’d forgotten it: you need to sum along the shallow diagonals (the lowest four blue squares make such a diagonal, where the sum is 21).

At morning tea, I noticed that the cold drink jugs seemed to have an elliptical cross-section. I wondered if they were really ellipses.

By the end of the day I had my answer: I rotated the photo (just because I like my longer axis parallel to the x-axis), and imported the cropped centre jug into Geogebra, centred it on the xy-axes, and tested the elliptical curve based on the length of the long and short axes. The ellipse (x/3.05)^2+(y/2.1)^2=1 fitted beautifully, as seen below in green.

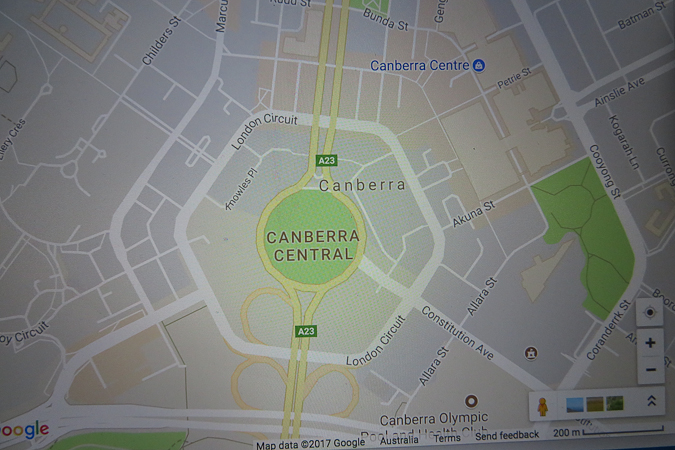
I needed to find an ATM at lunchtime, and so went to google maps where I noticed that central Canberra is nice and mathsy. If the diameter of the Canberra Central circle is 1/3 of the diameter of the surrounding London Circuit hexagon, what are their relative areas? (I haven’t actually calculated this, but know that I can.)

Between sessions, I noticed that there were some curved walls at the convention centre. They looked like they are circular, but I wondered how you would tell, and, if they are, how would you work out the diameter? What would you have to (and be able to) measure? (I’m not sure I can answer this. I think I could for the outside wall (the white ceiling where it meets the windows), but I’m less sure about the black wall.)

At lunch we had hexagonal prisms for dessert, which seemed entirely appropriate. There are plenty of maths questions that could be asked about this, but I was more inspired to ask the culinary question: how did they do that (as in, cut it so neatly)?

On my way to an afternoon session I walked up to the next level and took this photo of the stairs. For this situation I started to wonder if there is there enough information in the photo alone to work out anything at all about the measurements on the stair. I need to think about this one some more. Clearly my position as photographer has an influence, and we can’t get actual real-world length measurements, but if we think about ratios — and that ratios are not affected by scaling — maybe we can get somewhere. I’m not sure yet, though!

In the late afternoon some of us went on an excursion to Questacon, a big science museum in Canberra. Here I did the free-fall plunge, where you free-fall for a split second before landing on the slide and coming to a gentle stop at the bottom. What shaped curve works well for a free-fall slide in order to allow free-fall and cause no injuries? Is it a near-hyperbola?

At this point I realised that I had reached 12 photos, but there were still a couple of interesting mathsy things to do. At Questacon there was also a cross-shaped air-hockey table for four players. With all those extra edges and angles, what multi-bounce shots will work? Can you score around a corner? (There’s a spinning obstacle in the middle of the board, just to complicate matters, but you can ignore that for simplicity if you would like!)

One of the other activities at Questacon had been set up especially for our group of maths teachers, where six of us were given hand-bells of different pitches, and we walked through a sort of maze/network, which allowed us to meet in pairs and make comparisons to find which one of our two bells was the higher pitched. By the time we had worked our way to the end of the network, the decision tree/sorting algorithm that underpinned the maze/network meant that we had been sorted into order from lowest pitch at one end to highest at the other end.

So that was my mathsy day, in 14 (plus 1) rather than 12 photos, and rather too many words! Solutions to any or all problems can be submitted in the comments.

[The “12 of 12” project involves taking 12 photos on the 12th of the month. This provides the opportunity to get snapshots of different aspects of your life. I have been doing this since 2009 and this is the 95th such entry.]